Simulation of acoustic waves in complex media

This page displays the different studies that I have been working on under the topic of simulations of acoustic waves in complex media:

• Equivalent RLC Circuit Filtering for Implementing Acoustic Metamaterials in FDTD Schemes
• Geometrical Acoustic Modelling for Architectural Simulations

Equivalent RLC Circuit Filtering for Implementing Acoustic Metamaterials in FDTD Schemes

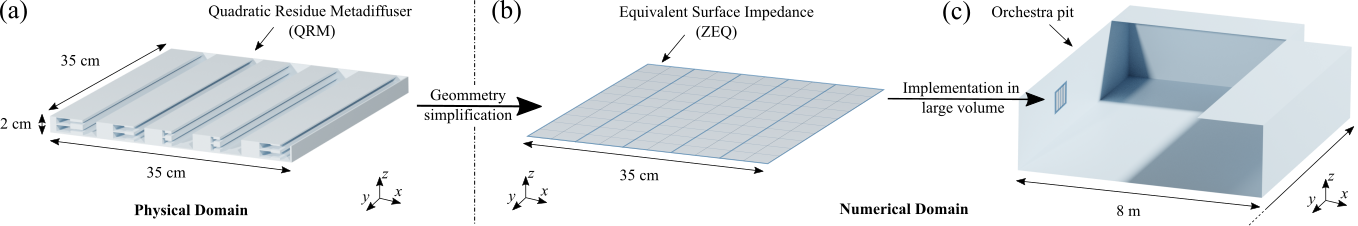
Most simulations involving metamaterials often require complex physics to be solved through refined meshing grids. However, it can prove challenging to simulate the effect of local physical conditions created by said metamaterials into much wider computing sceneries due to the increased meshing load. This study thus presents a framework for simulating complex structures with detailed geometries, such as metamaterials, into large Finite-Difference Time-Domain (FDTD) computing environments by reducing them to their equivalent surface impedance represented by a parallel-series RLC circuit. This reduction helps to simplify the physics involved as well as drastically reducing the meshing load of the model and the implicit calculation time. Here, an emphasis is made on scattering comparisons between an acoustic metamaterial and its equivalent surface impedance through analytical and numerical methods. Additionally, the problem of fitting RLC parameters to complex impedance data obtained from transfer matrix models is herein solved using a novel approach based on zero crossings of admittance phase derivatives. Despite the simplification process, the proposed framework achieves good overall results with respect to the original acoustic scatterer while ensuring relatively short simulation times over a vast range of frequencies.

Scattering Evaluation of Equivalent Surface Impedances of Acoustic Metamaterials in Large FDTD Volumes Using RLC Circuit Modelling,
E. Ballestero, B. Hamilton, N. Jiménez, V. Romero-García, J.-P. Groby, H. Aygün, S. Dance,
Appl. Sci., 11(17) 8084, 2021.

Comparison of waved-based simulations (via PFFDTD) of acoustic propagation between two versions of the same orchestra pit. (Top) Top view of an orchestra pit with rigid boundaries. (Bottom) Top view of an orchestra pit with traditional sound diffusers at the boundaries. N.B: Note how the diffusers help disribute the sound field in the orchestra pit much more rapidly than in the absence of diffusers. This usually can translate in better musical conditions for performing musicians as they sought a feeling of ensemble with respect to their own instrument's sound when palying.

Geometrical Acoustic Modelling for Architectural Simulations

Less precise than wave-based simulation techniques but generally much quicker, geometrical acoustics can be a strong tool when it comes to calculating virtually the acoustics of a place. This technique consists in assuming that the propagation of sound can be described to some extent as being similar than the one of light rays (assuming the case of very small wavelengths compared to physical obstacles), where such rays would bounce on any surface like billiard balls would, losing more or less energy as they travel like so depending on the obstacles' acoustical properties.

There are two main methods for solving geometrical acoustic problems: (i) image-source and (ii) ray-tracing. The former consists in determining the shortests paths of sound propagation between a sound source and one or more receivers. This involves calculating the image-sources of the principal sound source in order to determined the angles of sound reflection at the boundaries of the space. The latter method consists in launching a relatively large number of 'rays' emanating from the source in an omnidirectional way. Then, each ray would propagate in a straight line and bounce off the bounadries. ultimately, the energy of each sound ray passing through the receiver is collected. Of course, a mix of the two methods can be used and is generally performed, giving more accurate results.

The advent of parallel architectures, most notably through the use of Graphics Processing Units (GPUs), has allowed better efficiency for wave-based methods such as FEM or FDTD to become more rapid in their execution. Yet, for the time being, geometrical acoustic techniques still are the quickest way to simulate large architectural spaces with relative accuracy.